Mathematics for aliens
Can mathematics be communicated without words and symbols, for example in the form of a three-dimensional object? This was the challenge set for school students in the Space Competition run by the Department of Mathematics.
Schools from all over Switzerland responded to the challenge, submitting a total of 49 entries. The three-dimensional objects were displayed in the main hall of ETH Zurich from 11 to 22 March 2019 as part of the goMATH - going the MATH way exhibition. A jury of five mathematicians from the department judged the entries, praising the creativity and sophisticated mathematical messages they conveyed.
In this interview, Dr Kaloyan Slavov – jury member and originator of the challenge – describes how the competition came about and how the jury chose the winners.
The interview is followed by a description of the winning entries.
Do you remember how you came up with the idea for the competition?
The project team asked me to design a challenge that would require participants to produce an object with artistic merit that could be evaluated as part of a competition. They wanted the challenge to be accessible to a broad student audience, and I was keen for it to have a mathematical component. Initially I couldn’t think of anything, and I even wondered whether such a task was even possible! But eventually inspiration struck and I came up with the idea for the Space Competition.
Why did you choose the topic of space and aliens?
I was inspired by the Golden Records produced for the Voyager spacecraft. I could see how something like that could also be regarded as a work of art. Personally, I think mathematics is closer to arts and humanities than it is to science and technical disciplines. In mathematics, we create new things in addition to merely observing existing facts. We start with a blank piece of paper and write things down that didn’t exist before. So this exhibition was a good opportunity to promote my broad philosophical assertion that mathematics has an artistic dimension.
The schools that submitted entries were supported by students and doctoral students from the Department of Mathematics. Why do you think this support is necessary?
It offers an opportunity to connect schools with ETH through our students and get kids inspired and involved in the project. Our students led workshops in some of the schools, asked the pupils what they planned to do and gave them feedback. It also gave the school students a chance to learn some more mathematics.
As a member of the jury, you helped develop the competition criteria and judged the entries on that basis. Which criteria did the jury consider to be most important?
The most important thing was the clarity of the message. If you are trying to communicate mathematics without words or formulas, the message has to be very clearly presented. Before I put forward this challenge for the competition, I had already seen a few examples of 'proofs without words', but even the very best examples on the internet were inferior to the creations the students came up with! All three winners did an amazing job. Even if you are not familiar with the statements, you can figure them out – not by reading anything, but simply by interacting with the objects. That is truly remarkable.
We also evaluated the complexity of the message. If students shoot too high and aim for something too advanced, they risk the message not being understood. So they had to find the perfect balance. That’s a useful skill the students gained from this competition.
A further criteria was how they conveyed their concept of the proof. In mathematics, proofs are typically written in natural language, but here we challenged the students to convey a proof solely by means of an image. The task was designed to be accessible to students of various ages, so younger students stood much the same chance as older ones. We were delighted by how many schools took part.
What do you think of the quality of the entries?
I was really excited to see them and had no idea what to expect! I was genuinely impressed when I saw the results.
What particularly impressed you about the three winning objects?
The first object shows the centre of mass demonstrated by many examples of plain figures that can be laid on a pin to balance. You can see how the centre of mass is obtained as intersection of special lines. It is a very creative approach to communicate something by varying it. The production of the object was genius.
The second object shows that in a triangle any point of a hemisphere has an angle of 90 degrees when two points are diametrically opposite of a sphere. If they had picked just one fix point on the hemisphere there you could see the message, but just see a triangle. But as you move the point you can see that something stays the same: the 90 degree angle. This is represented in a really genius way. This is as clear as it can be.
The third object what representing the fact that some of the triangles have an angle sum of 180 degrees by using clocks. This was absolutely amazing. With the clocks the message is just so clear. The engineering of this third object was completely impressive.
All three winners have one thing in common: they produced not only one fix object, but a variable object that represent many states of something. If you show infinitely many things that obviously share a feature of the same family an alien will be able to understand the message much easier.
How important do you think these competitions are for promoting young talent?
One of our goals in running this competition was to communicate our own 'message without words', namely that there is a lot of mathematics going on outside the regular school curriculum! Mathematics is an open system: it is open for exploration, challenging, interesting and intellectually engaging. This competition exposed students to unconventional ways of thinking – and that’s exactly what mathematics is. It is not a routine chore of solving problems by following rules. Mathematics is a creative and intellectual pursuit that can be genuinely fun to explore.
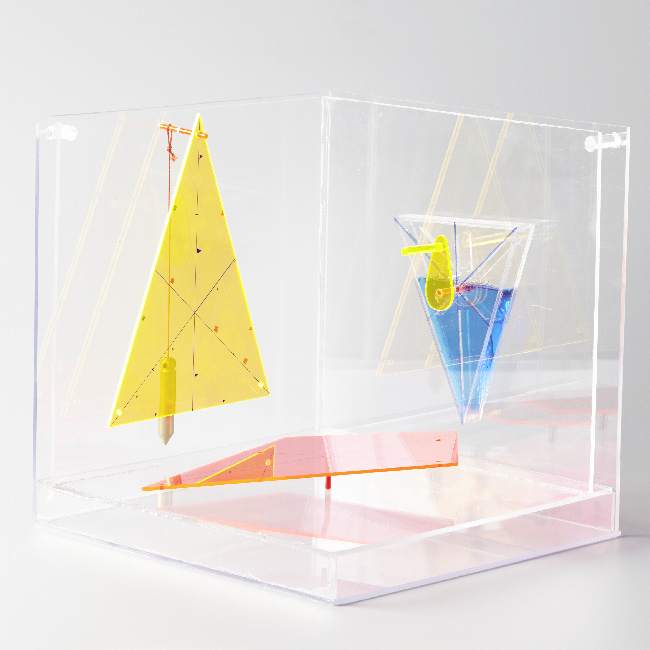
Object 49 is a beautiful toolkit for exploring properties of planar shapes. For example, it demonstrates that the centre of mass is both the balancing point and the unique point where certain lines intersect. In addition, it creatively shows how a median of a triangle divides the triangle's area in half. The jury praised the exceptional versatility of the object, the clear encouragement to experiment, and the efficacy of the presentation.
Second place
Object 43 demonstrates Thales' theorem in three dimensions, applying this famous theorem to show how any point on a sphere forms a right angle with two diametrically opposite points located at the equator. The jury praised the idea of extending this well-known circle theorem to a sphere. They were also impressed by the clear visual message and the way in which the object invites people to explore and interact with this theorem.
Third place
Object 38 is an animated, visual proof of a well-known theorem in geometry: the three angles of a triangle combine to form a semicircle. The jury was impressed by the clarity of the message, the sophisticated way of using motion to prove a geometric theorem, and the beautiful execution.
Audience award
Object 46 was the public’s clear favourite. It consists of two detailed flip books about the inscribed angle theorem and the special case of this theorem known as Thales' theorem. Rapidly flicking through the pages creates an animated effect that illustrates the concepts very nicely.